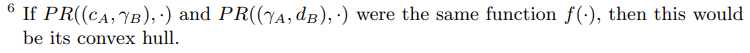How to Maximize Persuasion Ratios in Two-Phase Trials

Table of Links

Abstract and 1. Introduction

2. Problem Formulation

2.1 Model of Binary-Outcome Experiments in Two-Phase Trials

3 Binary-outcome Experiments in Two-phase Trials and 3.1 Experiments with screenings

3.2 Assumptions and induced strategies

3.3 Constraints given by phase-II experiments

3.4 Persuasion ratio and the optimal signaling structure

3.5 Comparison with classical Bayesian persuasion strategies

4 Binary-outcome Experiments in Multi-phase trials and 4.1 Model of binary-outcome experiments in multi-phase trials

4.2 Determined versus sender-designed experiments

4.3 Multi-phase model and classical Bayesian persuasion and References

3.4 Persuasion ratio and the optimal signaling structure

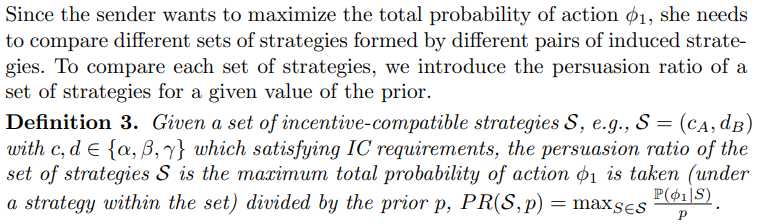
Careful readers may notice that if we multiply the persuasion ratio with the prior, the value will be the (maximum) expected utility the sender can achieve from the given set of strategies. Since the sender’s expected utility is monotone increasing in the prior p regardless of which set of strategies the sender adopts, the persuasion ratio under a given prior can be viewed as the relative utility gain this set of strategies can offer to the sender. Hence, given a specific prior, if a set of strategies has a higher persuasion ratio with respect to another set of strategies, the sender should use a strategy in the former instead of the latter.

According to this discussion, we can draw a persuasion ratio curve for each set of strategies as the prior is varied in [0, 1]. Abusing notation, we represent the persuasion ratio curve by P R(S). It may appear that an optimization needs to be carried out for each value of the prior. However, structural insights presented in the following two lemmas considerably simplify the analysis. Properties presented in Lemma 3 narrow down the space where the sender needs to search for the optimal signaling strategy. This allows us to depict persuasion ratio curves P R(S) for some basic strategies. On top of that, Lemma 4 provides a systematic approach to derive persuasion ratio curves for all types of strategies.

Lemma 3. Given a type of strategy S and a prior p, there exists a (sender’s) optimal strategy S ∈ S which satisfies one of the following two conditions:

1. At least one IC requirement of the constituent induced strategies is tight;

2. There is a signal that will be sent with probability 1 under S.

Before discussing the simplifications that Lemma 3 yields in terms of the key properties for solving the problem, we give an intuitive outline of the proof of Lemma 3. When the IC requirements of the two induced strategies are not tight and both signals are sent with non-zero probability in a strategy S, the sender can increase her expected utility by slightly raising the probability of the signal with a higher persuasion ratio (and adjust the probability of the other signal to respect the prior) to form a strategy S+. The sender can keep doing this ‘slight’ modification of her strategies until either one of the IC conditions is satisfied or the signal is sent with probability one.

Lemma 5. The persuasion ratio curve of the optimal signaling strategy P R∗ (p) is the upper envelope of the different types of strategies’ persuasion curves. Further, the optimal signaling strategy (under a given prior) is the strategy that reaches the frontier of the persuasion ratio curve (at that prior).

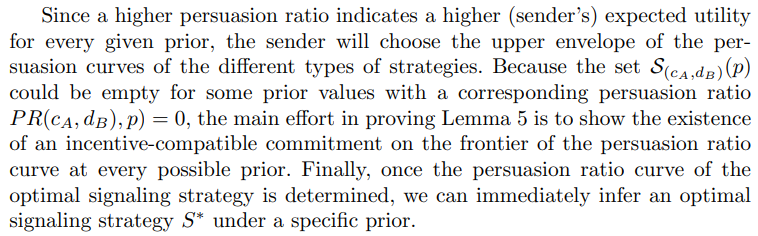
For a two-phase trial or to solve the last two phases of a trial with more than two phases studied in Section 4, the following corollary can further simplify the sender’s optimization procedure.

With Corollary 1, the Equation (3) in Lemma 4 reduces to a linear equation. Hence, the comparison in Lemma 5 and the computation in Lemma 4 can be reduced to a comparison of the (unique) corresponding IC strategies (if one exists) under the interim belief listed in Corollary1 for different types of strategies.